1. INTRODUCTION
In recent years, Korea's forest growing stock accumulation has been on a continuous increase, and efficient use of wood and development of technology are being requested. As is well known, wood is an anisotropic material that exhibits many differences in physical and mechanical properties in the longitudinal direction and perpendicular direction of the grain. This property shows many restrictions on use when using wood as a building material, and it is difficult to use it as a wide material. Therefore, plywood, which has markedly reduced anisotropy of wood by cross-laminating the grain directions of thin veneers, has played many roles in using wood as a wide plate material. However, it is becoming difficult to obtain large diameter hardwood, which has been widely used for the manufacture of veneers such as plywood and LVL, due to the recent forest damage caused by reckless logging and the protection of resources, and accordingly, the development of wooden boards for replacing plywood using small- and medium-diameter woods which can be obtained relatively easily, is actively progressing. Among them, cross-laminated timber (CLT) has been developed in Europe in recent years and has been widely used as a panel material with reduced anisotropy like plywood through various physical and mechanical performance studies (Byeon et al., 2018; Kang et al., 2019; Jang and Lee, 2019; Park et al., 2020; Galih et al., 2020; Choi et al., 2020; Choi et al., 2021). It is thought that it can be used efficiently in the forest environment of Korea, where the ratio of small- and medium- diameter woods is large.
Non-destructive evaluation of wood can be tested with a relatively easy test method and high reliability without damaging the wood, so various methods such as vibration method, acoustic emission (AE), acoustic ultrasonic (AU), and machine stress rated (MSR) are available for wood and wood-based materials (Tonosaki et al., 1983; Sobue and Nakano, 1984; Cha, 1996; Jang, 2000; Ayarkwa et al., 2001; Byeon et al., 2005; Park and Byeon, 2006, Hidayati et al., 2019). Among them, the flexural vibration method by the forced vibration of the magnetic driver is widely used as a non-destructive evaluation method for wood and wood-based materials because it is easy to test and has excellent test results.
In a previous study (Park et al., 2016), as a result of investigating the static bending strength performance of cross-laminated wood panels made with six temperate wood species, the bending strength performance increased with the increase of the density of the species regardless of the face lamina. It was found that the bending strength performance of the cross-laminated wood panels with perpendicular direction laminae in the faces was markedly improved by cross- laminating. In addition, the ratio between the calculated value and the measured value showed a remarkable difference in the cross-laminated wood panels with longitudinal direction laminae in the faces, and it was confirmed that the difference was greater in softwoods than in hardwoods.
In this study, for the purpose of efficient use and expansion of use of domestic softwoods and hardwoods, the dynamic characteristics of cross-laminated wood panels were revealed. And at the same time, in order to prepare an index for predicting non-destructive strength performance using dynamic MOE, cross- laminated wood panels were prepared using seven species of domestic softwoods and hardwoods. And the effect of the density of the species on the dynamic MOE of cross-laminated wood panels was investigated.
2. MATERIALS and METHODS
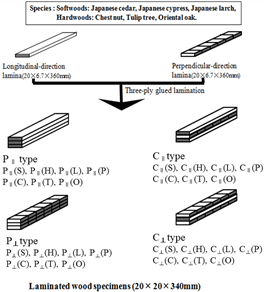
Seven species of temperate softwoods and hardwoods were selected for this study. They included four softwoods: Japanese cedar (density: 400 kg/m3), Japanese cypress (density: 452 kg/m3), Japanese red pine (density: 601 kg/m3) and Japanese larch (density: 604 kg/m3); three hardwoods: chestnut (density: 582 kg/m3), tulip tree (density: 595 kg/m3) and oriental oak (density: 901 kg/m3). Longitudinal-direction laminae of 6.7 (T) × 20 (R) × 360 (L) mm whose long axes were parallel to the grain were made with seven species. Elements of 7.5 (T) × 20 (R) × 180 (L) mm were cut from each seven species, 18 elements from each species were edge-jointed and then cut to 20 mm size in width direction and perpendicular-direction laminae of 6.7 (T) × 360 (R) × 20 (L) mm were made with long axes perpendicular to the grain. The annual ring angles of both laminae were 90◦. An isocyanate type adhesive (MPU-500) formulated for a room temperature cure was used, and the amount of spread was 300 g/m2. The three-ply laminae were pressed under pressure of 0.34 MPa for 24 hours in a room maintained at 20℃ and 65%RH. Fig. 1 shows three-ply parallel- and cross-laminated wood panel specimens tested. P∥type (P∥(S), P∥(H), P∥(P), P∥(L), P∥(C), P∥(T) and P∥(O), where S: Japanese cedar, H: Japanese cypress, P: Japanese red pine, L: Japanese larch, C: Chestnut, T: Tulip tree, O: Oriental oak) and P⊥type (P⊥(S), P⊥(H), P⊥(P), P⊥(L), P⊥(C), P⊥(T) and P⊥(O)) were the specimens used to measure the bending strength performances parallel and perpendicular to the grain of parallel-laminated wood panels, respectively. C∥ type (C∥(S), C∥(H), C∥(L), C∥(P), C∥(C), C∥(T) and C∥(O)) and C⊥ type (C⊥(S), C⊥(H), C⊥(P), C⊥(L), C⊥(C), C⊥(T) and C⊥(O)) were the specimens used to measure the bending strength performances parallel and perpendicular to the grain of the face laminae of cross-laminated wood panels, respectively. Using a total of seven species of four softwood and three hardwoods, 28 types of parallel- and cross-laminated wood panels were manufactured. A total of 280 laminated specimens, 10 for each type, were prepared to investigate the dynamic behavior.
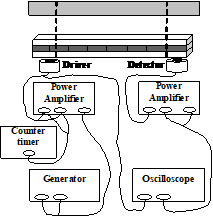
Vibration was induced via a small steel plate attached to the bottom end of 3-ply laminated wood specimens and suspended by two threads at the magnetic driver as shown in Fig. 2. The vibration was received at a small steel plate attached to the other end. The test was made with both ends free. The test apparatus consisted of a sine wave generator (Type 1023, B&K), a universal counter timer (Type 5001, GSP) and an oscilloscope (Type 1740A, HP). The value of the frequency counter timer was recorded when the relative amplitude indicated the highest value on the oscilloscope. Resonance frequency ( f ) and dynamic MOE (MOEd) were calculated by the following equations (Kataoka and Ono, 1975):
where f0 is the value obtained from frequency counter timer, α= 8.2 isthe value according to chosen vibration type, h isthe thickness of specimen (mm), l is the length of specimen (mm).
where ρ is density (kg/m3), m = 4.73 isthe value according to the chosen basic vibration, h isthe thickness of specimen (mm), l is the length of specimen (mm).
3. RESULTS and DISCUSSION
Results of the flexural vibration test under free-free beam condition of parallel- and cross-laminated wood panels made with four softwoods and three hardwoods are shown in Table 1. And Fig. 3. shows the effect of the density on the dynamic MOE of the laminated wood panels.
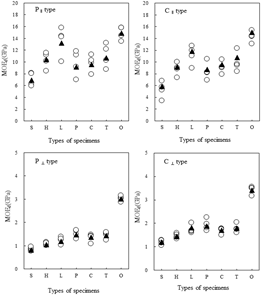
As shown in Fig. 3, in the case of the parallel-laminated wood panel of P∥ type, the P∥(O) type which all three layers were composed with oriental oak showed the highest value at 14.9 GPa. P∥(S) type which all three layers were composed with Japanese cedar showed the lowest value at 6.96 GPa. These values generally showed an increase in the dynamic MOE with an increase in the density of the species, indicating the same density dependence as the static bending MOE (Park et al., 2016). However, the P∥(P) type which composed of red pine showed relatively low dynamic MOE compared to the density due to much resin and not straight grain. The value was in order of P∥(O)>P∥(L)>P∥(T)>P∥(H)>P∥(C)>P∥(P)>P∥(S), and it was similar to that of static bending MOE. This value was almost equal to or slightly higher by 0.96 to 1.12 times than the static MOE.
As shown in the lower left of Fig. 3, in the case of P⊥ type parallel laminated wood panels, P⊥(O) type composed of all three layers of oriental oak showed a value of 3.01 GPa, showing the highest value. The P⊥(S) type, where all layers were composed of Japanese cedar, showed the lowest value of 0.829 GPa. These values showed an increase in the dynamic MOE with an increase in the density of the species. This value was significantly lower by 0.09 to 0.20 times than that of the P∥ type parallel laminated wood panels. The value is in order of P⊥(O)>P⊥(P)>P⊥(T)>P⊥(C)>P⊥(L)>P⊥(H)>P⊥(S). It was confirmed that the density dependence was higher than that of the P∥ type composed of longitudinal-direction laminae in all three layers. This value was 1.04 - 1.14 times that of the static MOE, which was slightly higher than that of the static MOE. This was consistent with Norimoto's research that dynamic MOE of wood had a higher value of 1.1-1.2 times that of static MOE (Norimoto, 1982).
As shown in the upper right of Fig. 3, in the case of C∥type which was composed of perpendicular-direction laminae in the core and longitudinal-direction laminae in the faces, C∥(O) type with oriental oak longitudinal- and perpendicular-direction laminae in the faces and the core showed the highest value of 14.4 GPa. The C∥(S) type with Japanese cedar longitudinal- and perpendicular-direction laminae in the face and the core showed the lowest value of 5.21 GPa. This value showed a low value of 0.75-0.97 times of P∥type. The values were in the order of C∥(O)>C∥(L)>C∥(T)>C∥(C)>C∥(H)>C∥(P)>C∥(S), and overall, it was confirmed that the dynamic MOE increased with increasing density of the species. However, It was confirmed that in the case of the C∥(P) type composed of the face and the core of red pine longitudinal- and perpendicular-direction laminae, the value showed a lower value compared to the density of the species like the P∥type. It was confirmed that this value was 0.93-1.04 times the value of the static MOE, which was almost the same as or slightly higher than that of the static MOE. And it was also slightly lower than the P∥type.
As shown in the lower right of Fig. 3, in the C⊥ type cross-laminated wood panel which was composed of longitudinal-direction laminae in the faces and perpendicular-direction lamina in the core, the C⊥(O) type with oriental oak in the faces and the core has the highest value of 3.42 GPa. The C⊥(S) type with Japanese cedar in the faces and the core showed the lowest value of 1.20 GPa. Like the P⊥ type parallel laminated wood panels, this value increased in proportion to the density of the species, and the density dependence showed a clearer tendency than that of the P∥ type. The dynamic MOE was 1.3 to 1.5 times higher than that of the P⊥ type by arranging longitudinal- direction lamina in the core of the P⊥ type with perpendicular-direction laminae in all three layers. The value was in order of C⊥(O)>C⊥(P)>C⊥(L)>C⊥(T)>C⊥(C)>C⊥(H)>C⊥(S), and according to density increase, the change of the dynamic MOE showed a similar tendency to that of the P⊥ type, but the extent of the increase was slightly lower than that of the P⊥ type. It was confirmed that this value represents a high value of 2-11% of the static MOE, which was almost consistent with the study of Norimoto (1982).
The degree of anisotropy for the laminated wood panels expressed as the ratio of the dynamic MOE perpendicular to the grain of face laminae versus that parallel to the grain of the face laminae was Japanese cedar 1: 0.119 to 0.230, Japanese cypress 1: 0.101 to 0.165, Japanese larch 1: 0.090 to 0.164, Japanese red pine 1: 0.162~0.228, chestnut 1: 0.143~0.189, tulip tree 1: 0.136~0.191, oriental oak 1: 0.202~0.238. It was found that the anisotropy significantly decreased by cross-laminating.
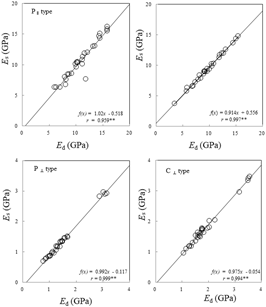
Least squares regression analyses were conducted to examine the relation dynamic MOE by flexural vibration and static bending strength performances, and the derived regression parameters are summarized in Table 2. The relation between the dynamic MOE and static bending MOE of parallel- and cross-laminated wood panels is shown in Fig. 4. In both parallel-laminated wood panels of P∥and P⊥ types and cross-laminated wood panels of C⊥and C∥types, a very strong correlation with a significance level of 1% was found between the dynamic MOE and the static bending MOE. The correlation coefficient showed very high values of 𝑟 = 0.959 in P∥ type, 𝑟 = 0.999 in P⊥ type, 𝑟 = 0.997 in C∥ type, and 𝑟 = 0.994 in C⊥ type. This results agreed with various previous researches for solid wood and wood-based materials which there was a high correlation between dynamic MOE and static MOE (Mastumoto and Tsutsumi, 1968; Norimoto, 1982; Bender et al., 1990; Lee et al., 1997; Ayarkwa et al., 2001; Park et al., 2004; Park and Byeon, 2006; Byeon et al., 2005a). This result indicated that the static bending modulus could be predicted nondestructively through the dynamic MOE.
The relationship between dynamic MOE and bending proportional limit stress of parallel- and cross- laminated wood panels is shown in Fig. 5. In both parallel-laminated wood panels and cross-laminated wood panels, a very strong correlation with a significance level of 1% was found between the dynamic MOE and the static bending proportional limit stress like the relation between dynamic MOE and static bending MOE. The correlation coefficient showed very high values of 𝑟 = 0.912 in P || type, 𝑟 = 0.899 in P⊥ type, 𝑟 = 0.964 in C∥ type, and 𝑟 = 0.865 in C⊥ type. From this result, it was confirmed that the static bending proportional limit stress could be predicted non-destructively through the dynamic MOE.
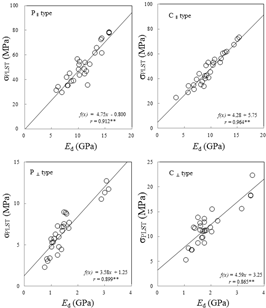
Fig. 6 shows relationship between dynamic MOE and static bending strength of parallel- and cross-laminated wood panels. There was a strong correlation between dynamic MOE and static bending strength like the relations between dynamic MOE and static bending MOE. The correlation coefficient showed very high values of 𝑟 = 0.852 in P∥ type, 𝑟 = 0.803 in P⊥ type, 𝑟 = 0.923 in C∥ type, and 𝑟 = 0.769 in C⊥ type. It was found that the extent of the relation was stronger in the laminated wood panels with longitudinal- direction laminae in the faces than in that with perpendicular-direction laminae in the faces. This value was higher than the result of a previous study (Park et al., 2011) on the prediction of the bending strength performance of hybrid board composed of green tea and wood fiber by flexural vibration, and was similar to previous study for the laminated wood panels composed of Japanese cedar and beech longitudinal-direction laminae and five species perpendicular-direction laminae (Park and Byeon, 2006). Also, this result agreed with various previous reports which there was a high correlation both dynamic MOE and static bending strength for the wood and wood-based materials (Nakayama, 1975; Bender et al., 1990; Ayarkwa, 2001; Park et al., 2004; 2006; Byeon et al., 2005a). Although the correlation coefficient between the dynamic MOE and the static bending strength was lower than those between dynamic MOE and the static bending MOE or the static bending proportional limit stress, high significance was recognized at the reliability level of 1%. From this result, it was found that static bending strength could be predicted non-destructively through the dynamic MOE like static bending MOE.

4. CONCLUSION
1. In all P∥ type and P⊥ type parallel laminated wood panels and C∥ type and C⊥ type cross- laminated wood panels, the dynamic MOE had the highest value in the type composed of oriental oak and had the lowest value in the type composed of Japanese cedar.
2. Dynamic MOE showed the density dependence in all laminated wood panels on the whole, the extent of the density dependence was greater in P⊥ and C⊥ type with perpendicular-direction laminae in the faces than in P∥ and C∥ type with longitudinal-direction lamina in the faces.
3. In all types of parallel- and cross-laminated wood panels, there was a very strong correlation between the dynamic MOE and the static bending MOE at a significance level of 1%, and it was confirmed that the static bending MOE could be predicted nondestructively using the dynamic MOE.
4. In all types of parallel- and cross-laminated wood panels, a significance level of 1% was recognized between the dynamic MOE and the static bending proportional limit stress or the static bending strength, and both of them showed a close correlation with the dynamic MOE, so it is considered that the static bending proportional limit and static bending strength could be predicted non-destructively using the dynamic MOE.
